Circles

The chapter on Circles introduces the fundamental concepts related to circles in geometry.

Key Topics

• Basic Definitions: Circle, center, radius, diameter, chord, secant, and tangent.
• Properties of Chords: Relationship between chords and their distances from the center.
• Angles in a Circle: Angles subtended by arcs, chords, and segments.
• Cyclic Quadrilaterals: Properties of quadrilaterals inscribed in a circle.

Core Ideas

• Understanding the different components of a circle and their relationships.
• Exploring the properties of chords and their distances from the center.
• Analyzing the relationship between angles and arcs in a circle.
• Recognizing the properties of cyclic quadrilaterals.

This chapter provides a foundation for more complex geometric concepts related to circles.

Exercise 9.1

1. Recall that two circles are congruent, if they have the same radii. Prove that equal chords of congruent circles subtend equal angles at their centres

Ans : 

Given: Two congruent circles with centers O and O’, and equal chords AB and PQ.

To Prove: Angle AOB = Angle PO’Q

Proof:

1. Draw radii: Join OA, OB, O’P, and O’Q.
2. Congruent Triangles:
   • Therefore, OA = O’P and OB = O’Q.
   • Given that AB = PQ.
   • Hence, by the Side-Side-Side (SSS) congruence criterion, triangle AOB is congruent to triangle PO’Q.
3. Corresponding Parts of Congruent Triangles:
   • Since triangles AOB and PO’Q are congruent, their corresponding parts are equal.
   • Therefore, angle AOB = angle PO’Q.

2. Prove that, if chords of congruent circles subtend equal angles at their centres, then the chords are equal.

Ans : 

Given: Two congruent circles with centers O and O’, and chords AB and PQ such that angle AOB = angle PO’Q.

To Prove: AB = PQ.

Proof:

1. Join the centers to the endpoints of the chords: Draw radii OA, OB, O’P, and O’Q.
2. Congruent Triangles:
   • Therefore, OA = O’P and OB = O’Q.
   • Given that angle AOB = angle PO’Q.
   • Hence, by the Side-Angle-Side (SAS) congruence criterion, triangle AOB is congruent to triangle PO’Q.
3. Corresponding Parts of Congruent Triangles:
   • Since triangles AOB and PO’Q are congruent, their corresponding parts are equal.
   • Therefore, AB = PQ.

Exercise 9.2

1. Two circles of radii 5 cm and 3 cm intersect at two points and the distance between their centres is 4 cm. Find the length of the common chord.

Ans : 

∴∠OLP = ∠OLQ = 90° and PL = LQ

Now, in right ∆OLP, we have

PL2 + OL2 = 2

⇒ PL2 + (4 – x)2 = 52

⇒ PL2 = 25 -16 – x2 + 8x

⇒ PL2 = 9 – x2 + 8x …(i)

Again, in right ∆O’LP,

PL2 = PO‘2 – LO‘2

= 3^2 – x^2 

= 9 – x^2 …(ii)

From (i) and (ii), we have

9 – x^2 + 8x = 9 – x^2

⇒ 8x = 0

⇒ x = 0

⇒ L and O’ coincide.

⇒ PL = 3 cm

But PL = LQ

∴ LQ = 3 cm

∴ PQ = PL + LQ = 3cm + 3cm = 6cm

2. If two equal chords of a circle intersect within the circle, prove that the segments of one chord are equal to corresponding segments of the other chord.

Ans :

To Prove:

• AE = CE and BE = DE

Proof:

Draw perpendiculars OM and ON from the center O to the chords AB and CD respectively, intersecting them at points M and N

.

1. Equal chords are equidistant from the center:
   • Since AB and CD are equal chords, their distances from the center are equal.
   • Therefore, OM = ON.
2. Right triangles OME and ONE:
   • Triangles OME and ONE are right-angled triangles with right angles at M and N respectively.
   • OM = ON (proved above)
   • OE is common to both triangles.
3. Congruence of triangles:
   • By the RHS (Right Angle – Hypotenuse – Side) congruence rule, triangle OME is congruent to triangle ONE.
4. Equal corresponding parts:
   • Since the triangles are congruent, their corresponding parts are equal.
   • Therefore, ME = NE.
5. Bisected chords:
   • The perpendicular from the center of a circle to a chord bisects the chord.
   • Therefore, AM = MB and CN = ND.
6. Equal segments:
   • Since AM = MB and ME = NE, we have AE = CE.
   • Similarly, since CN = ND and ME = NE, we have BE = DE.

Hence, AE = CE and BE = DE, proving that the segments of one chord are equal to the corresponding segments of the other chord.

3. If two equal chords of a circle intersect within the circle, prove that the line joining the point of intersection to the centre makes equal angles with the chords.

Ans : 

To Prove:

• The line joining the point of intersection E to the center O makes equal angles with the chords AB and CD. That is, ∠OEB = ∠OED.

Proof:

1. Draw perpendiculars from O to AB and CD:
   • Let OM and ON be the perpendiculars from O
   •  to AB and CD respectively.
2. Equal chords are equidistant from the center:
   • Since AB and CD are equal chords, their perpendicular distances from the center are equal.
   • Therefore, OM = ON.
3. Right-angled triangles OME and ONE:
   • Triangles OME and ONE are right-angled triangles with right angles at M and N respectively.
   • OM = ON (proved above)
   • OE is common to both triangles.
4. Congruence of triangles:
   • By the RHS (Right Angle – Hypotenuse – Side) congruence rule, triangle OME is congruent to triangle ONE.
5. Equal angles:
   • Therefore, ∠OEB = ∠OED.

Hence, the line joining the point of intersection E to the center O makes equal angles with the chords AB and CD.

4. If a line intersects two concentric circles (circles with the same centre) with centre 0 at A, B, C and D, prove that AB = CD (see figure).

Ans : 

Proof:

Step 1: Draw a Perpendicular from the Center

• Draw a perpendicular OM from the center O to the line AD, intersecting it at point M.

Step 2: Apply the Perpendicular Bisector Theorem

• In the larger circle, AD is a chord and OM is perpendicular to it.
• Therefore, OM bisects AD (property of a chord and its perpendicular bisector).
• So, AM = MD.

Step 3: Apply the Perpendicular Bisector Theorem to the Smaller Circle

• Similarly, in the smaller circle, BC is a chord and OM is perpendicular to it.
• Therefore, OM bisects BC.
• So, BM = CM.

Step 4: Subtract the Equal Segments

• Subtracting equation 2 from equation 1, we get:
  • AM – BM = MD – CM
  • This simplifies to AB = CD.

Conclusion:

Hence, we have proved that AB = CD.

5. Three girls Reshma, Salma and Mandip are playing a game by standing on a circle of radius 5m drawn in a park. Reshma throws a ball to Salma, Salma to Mandip, Mandip to Reshma. If the distance between Reshma and Salma and between Salma and Mandip is 6 m each, what is the distance between Reshma and Mandip?

Ans : 

RS = SM = 6 m [Given]

Equal chords of a circle subtend equal angles at the centre.

∴ ∠1 = ∠2

In ∆POR and ∆POM, we have

OP = OP [Common]

OR = OM

 [Radii of the same circle]

∠1 = ∠2 [Proved above]

∴ ∆POR ≅ ∆POM 

[By SAS congruence criteria]

∴ PR = PM and

∠OPR = ∠OPM [C.P.C.T.]

∵∠OPR + ∠OPM = 180° [Linear pair]

∴∠OPR = ∠OPM = 90°

⇒ OP ⊥ RM

Now, in ∆RSP and ∆MSP, we have

RS = MS [Each 6 cm]

SP = SP [Common]

PR = PM [Proved above]

∴ ∆RSP ≅ ∆MSP [By SSS congruence criteria]

⇒ ∠RPS = ∠MPS [C.P.C.T.]

But ∠RPS + ∠MPS = 180° [Linear pair]

⇒ ∠RPS = ∠MPS = 90°

SP passes through O.

Let OP = x m

∴ SP = (5 – x)m

Now, in right ∆OPR, we have

x2 + RP2 = 52

RP2 = 52 – x2

In right ∆SPR, we have

(5 – x)2 + RP2 = 62

⇒ RP2 = 62 – (5 – x)2 ……..(ii)

From (i) and (ii), we get

⇒ 52 – x2 = 62 – (5 – x)2

⇒ 25 – x2 = 36 – [25 – 10x + x2]

⇒ – 10x + 14 = 0

⇒ 10x = 14 ⇒ x = 

14

10

 = 1.4

Now, RP2 = 52 – x2

⇒ RP2 = 25 – (1.4)2

⇒ RP2 = 25 – 1.96 

= 23.04

∴ RP = 23.04 _____√= 4.8

∴ RM = 2RP = 2 x 4.8

 = 9.6

Thus, distance between Reshma and Mandip is 9.6 m.

6. A circular park of radius 20 m is situated in a colony. Three boys Ankur, Syed and David are sitting at equal distance on its boundary each having a toy telephone in his hands to talk each other. Find the length of the string of each phone.

Ans : 

Solution

Step 1: Visualize the Problem

 Imagine the three boys positioned equally on the circumference of the circle. The lines connecting them form an equilateral triangle.

Step 2: Find the side length of the equilateral triangle 

The side length of this equilateral triangle is equal to the diameter of the circle, which is twice the radius.

• Side length = 2 * radius = 2 * 20 m = 40 m

Step 3: Each boy holds one end of the string, so the length of the string is half the side length of the equilateral triangle.

• Length of string = side length / 2 = 40 m / 2 = 20 m

Exercise 9.3

1. In figure A,B and C are three points on a circle with centre 0 such that ∠BOC = 30° and ∠ AOB = 60°. If D is a point on the circle other than the arc ABC, find ∠ ADC.

Ans :

We have a circle with centre O, such that

∠AOB = 60° and ∠BOC = 30°

∵∠AOB + ∠BOC = ∠AOC

∴ ∠AOC = 60° + 30° = 90°

∴ ∠ ADC = 1/2 (∠AOC) = 1/2(90°) = 45°

2. A chord of a circle is equal to the radius of the circle, find the angle subtended by the chord at a point on the minor arc and also at a point on the major arc.

Ans : 

We have ,

∴ AO = BO = AB

⇒ ∆AOB is an equilateral triangle.

angle of an equilateral triangle is 60°.

⇒ ∠AOB = 60°

Since, the arc ACB makes reflex ∠AOB = 360° – 60° = 300° at the centre of the circle and ∠ACB

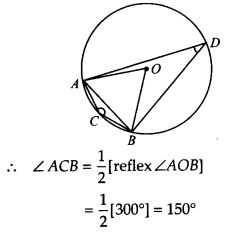
at a point on the minor arc of the circle.

Hence,  chord on the minor arc = 150°.

Similarly, ∠ADB = 12 [∠AOB] = 12 x 60° = 30°

Hence,  chord on the major arc = 30°

3. In figure, ∠PQR = 100°, where P, Q and R are points on a circle with centre O. Find ∠OPR.

Ans : 

∴ reflex ∠POR = 2∠PQR

But ∠PQR = 100°

∴ reflex ∠POR = 2 x 100° = 200°

Since, ∠POR + reflex ∠POR = 360°

⇒ ∠POR = 360° – 200°

⇒ ∠POR = 160°

Since, OP = OR [Radii of the same circle]

∴ In ∆POR, ∠OPR = ∠ORP

[Angles opposite to equal sides of a triangle are equal]

Also, ∠OPR + ∠ORP + ∠POR = 180°

[Sum of the angles of a triangle is 180°]

⇒ ∠OPR + ∠ORP + 160° = 180°

⇒ 2∠OPR = 180° -160° = 20° [∠OPR = ∠ORP]

⇒ ∠OPR = 20∘2 = 10°

4. n figure, ∠ABC = 69°,∠ACB = 31°, find ∠BDC.

Ans : 

• Find angle BAC:
  • In triangle ABC, the sum of angles is 180°.
  • So, ∠BAC + ∠ABC + ∠ACB = 180°
  • Substituting the given values, we get: ∠BAC + 69° + 31° = 180° ∠BAC + 100° = 180° ∠BAC = 80°
• Angles in the same segment:
  • Angles in the same segment of a circle are equal.
  • Therefore, ∠BDC = ∠BAC.

Hence, ∠BDC = 80°.

Therefore, the measure of angle BDC is 80 degrees.

5. In figure, A, B , C and D are four points on a circle. AC and BD intersect at a point E such that ∠ BEC = 130° and ∠ ECD = 20°. Find ∠BAC.

Ans : 

1. Finding ∠ABD:

• So, ∠ABD = ∠ECD = 20°

2. Finding ∠BAC:

• In triangle ABE, ∠BEC is an exterior angle.
• So, ∠BEC = ∠BAC + ∠ABD
• Substituting the known values, we get: 130° = ∠BAC + 20° ∠BAC = 130° – 20° = 110°

Therefore, ∠BAC = 110°.

6. ABCD is a cyclic quadrilateral whose diagonals intersect at a point E. If ∠DBC = 70°, ∠BAC is 30°, find ∠BCD. Further, if AB = BC, find ∠ECD.

Ans : 

1. Finding ∠BCD

• So, ∠DBC + ∠BAC = ∠BCD + ∠ADC = 180°.
• Substituting the given values, 
• we get: 70° + 30° = ∠BCD + ∠ADC 100° = ∠BCD + ∠ADC
• Since we only need to find ∠BCD, we don’t need the exact value of ∠ADC.

Therefore, ∠BCD = 100° – ∠ADC.

2. Finding ∠ECD (if AB = BC)

• So, ∠BAC = ∠BCA = 30°.
• In triangle BCE, ∠BEC = 180° – (∠EBC + ∠ECB) = 180° – (70° + 30°) = 80°.
• Since ∠ECD and ∠BEC are in the same segment, ∠ECD = ∠BEC = 80°.

Therefore, ∠ECD = 80°.

7. If diagonals of a cyclic quadrilateral are diameters of the circle through the vertices of the quadrilateral, prove that it is a rectangle.

Ans : 

1. Angles in a semicircle:

• Angles ∠ABD, ∠BCD, ∠ACD, and ∠BAD are angles in a semicircle.
• An angle in a semicircle is a right angle.
• Hence, ∠ABD = ∠BCD = ∠ACD = ∠BAD = 90°.

2. Properties of a rectangle:

• A quadrilateral with all angles equal to 90° is a rectangle.

Conclusion:

• Since all angles of quadrilateral ABCD are 90°, it is a rectangle.

8. If the non – parallel sides of a trapezium are equal, prove that it is cyclic.

Ans : 

Proof:

1. Congruent Triangles:

• In triangles AMD and BNC,
  • AD = BC (given)
  • ∠AMD = ∠BNC (both are right angles)
  • DM = BN (perpendicular distance between parallel lines)
• Therefore, by the RHS congruence rule, ΔAMD ≅ ΔBNC.

2. Equal Angles:

• Since triangles AMD and BNC are congruent, ∠MAD = ∠NBC.

3. Sum of Interior Angles:

• In trapezium ABCD, AB || DC. Therefore, ∠A and ∠D are interior angles on the same side of the transversal AD.
• So, ∠A + ∠D = 180°.

4. Substituting Equal Angles:

• Since ∠MAD = ∠NBC, we can substitute ∠NBC for ∠MAD in the equation ∠A + ∠D = 180°.
• This gives us ∠B + ∠D = 180°.

Conclusion:

• Since the sum of opposite angles (∠B and ∠D) in quadrilateral ABCD is 180°, ABCD is a cyclic quadrilateral.

Therefore, if the non-parallel sides of a trapezium are equal, it is cyclic.

9. Two circles intersect at two points B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A,D and P, Q respectively (see figure). Prove that ∠ ACP = ∠QCD.

Ans : 

Proof:

1. Angles in the same segment:
   • Angle ACP and angle ABP lie in the same segment of one circle.
   • Therefore, ∠ACP = ∠ABP.
   • Similarly, angle DCQ and angle DBQ lie in the same segment of the other circle.
   • Therefore, ∠DCQ = ∠DBQ.
2. Vertically opposite angles:
   • Angle ABP and angle DBQ are vertically opposite angles.
   • Therefore, ∠ABP = ∠DBQ.
3. Conclusion:
   • From steps 1 and 2, we have:
     • ∠ACP = ∠ABP
     • ∠DBQ = ∠DCQ
     • ∠ABP = ∠DBQ
   • Therefore, ∠ACP = ∠QCD.

Hence, ∠ACP = ∠QCD.

10. If circles are drawn taking two sides of a triangle as diameters, prove that the point of intersection of these circles lie on the third side.

Ans : 

Proof:

1. Angles in a semicircle:
   • Since AB is the diameter of one circle, angle ADB is an angle in a semicircle and hence is a right angle.
   • Similarly, since AC is the diameter of the other circle, angle ADC is also a right angle.
2. Linear Pair:
   • Angle ADB + angle ADC = 180°.
   • Substituting the values, we get: 90° + 90° = 180°.
3. Conclusion:
   • Since the sum of angles ADB and ADC is 180°, BDC is a straight line.
   • Hence, point D lies on the line segment BC.

Therefore, the point of intersection of the two circles lies on the third side BC of the triangle.

11. ABC and ADC are two right angled triangles with common hypotenuse AC. Prove that ∠CAD = ∠CBD.

Ans : 

Proof:

1. Cyclic Quadrilateral:
   • Since triangles ABC and ADC are right-angled, their right angles (angles B and D) add up to 180°.
   • Therefore, quadrilateral ABCD is a cyclic quadrilateral (as the sum of opposite angles is 180°).
2. Angles in the Same Segment:
   • Angles CAD and CBD are in the same segment CD.
3. Conclusion:
   • Therefore, ∠CAD = ∠CBD.

Hence, ∠CAD = ∠CBD.

12. Prove that a cyclic parallelogram is a rectangle.

Ans : 

• Property of a cyclic quadrilateral: The sum of opposite angles in a cyclic quadrilateral is 180°.
• Property of a parallelogram: Opposite angles of a parallelogram are equal.

Let ABCD be a cyclic parallelogram.

• Since ABCD is a parallelogram, 
• ∠A = ∠C and ∠B = ∠D.
• Since ABCD is cyclic, ∠A + ∠C = 180° and ∠B + ∠D = 180°.

Substituting ∠C for ∠A in the first equation, we get:

• ∠A + ∠A = 180°
• 2∠A = 180°
• ∠A = 90°

Since ∠A = ∠C and ∠B = ∠D, all angles of the parallelogram ABCD are 90°.

Therefore, a cyclic parallelogram is a rectangle.