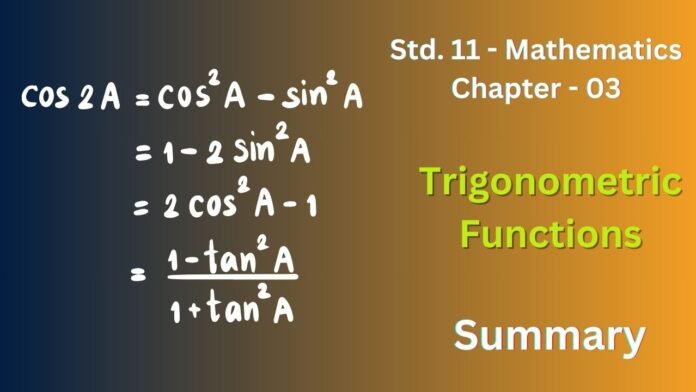
Chapter 3.1: Introduction to Trigonometry
- Definition: The study of relationships between the sides and angles of a triangle.
- Trigonometric Ratios: Sine, cosine, tangent, cotangent, secant, cosecant.
- Trigonometric Identities: Fundamental relationships between trigonometric functions.
Chapter 3.2: Trigonometric Functions
- Domain and Range: The set of possible input and output values for trigonometric functions.
- Graphs of Trigonometric Functions: Visual representation of the behavior of trigonometric functions.
- Periodic Functions: Functions that repeat their values after a fixed interval (period).
Chapter 3.3: Trigonometric Identities
- Sum and Difference Formulas: Formulas for trigonometric functions of the sum or difference of angles.
- Multiple Angle Formulas: Formulas for trigonometric functions of multiples of an angle.
Chapter 3.4: Trigonometric Equations
- Solving Trigonometric Equations: Finding the values of x that satisfy a given trigonometric equation.
- General Solution: Expressing the solution of a trigonometric equation in terms of the principal solution and the period of the function.
Key Concepts:
- Trigonometric ratios and their relationships
- Trigonometric functions and their graphs
- Trigonometric identities
- Solving trigonometric equations
This chapter provides a foundational understanding of trigonometry, which is essential for various mathematical and scientific applications.
Exercise 3.1
1. Find the radian measures corresponding to the following degree measures:
(i) 25° (ii) – 47°30′ (iii) 240° (iv) 520°
Ans :
o convert from degrees to radians, we multiply by π/180.
(i) 25° = 25 * (π/180) = 5π/36 radians
(ii) -47°30′ = -47.5° = -47.5 * (π/180) = -19π/72 radians
(iii) 240° = 240 * (π/180) = 4π/3 radians
(iv) 520° = 520 * (π/180) = 26π/9 radians
2.
Ans :
To convert from radians to degrees, we multiply by 180/π.
Given: π = 22/7
(i) 11π/16 = 11 * (22/7) * (180/π) = 11 * 22 * 180 / 7 = 5820/7 ≈ 831.43°
(ii) -4 = -4 * (180/π) ≈ -4 * 180 * 7/22 ≈ -229.09°
(iii) 5π/3 = 5 * (22/7) * (180/π) = 5 * 22 * 180 / 7 = 2828.57° ≈ 300°
(iv) 7π/6 = 7 * (22/7) * (180/π) = 7 * 22 * 180 / 7 = 2520° ≈ 210°
3. A wheel makes 360 revolutions in one minute. Through how many radians does it turn in one second?
Ans :
Given:
- The wheel makes 360 revolutions in one minute.
Steps:
- Convert minutes to seconds:
- 1 minute = 60 seconds
- Calculate revolutions per second:
- Revolutions per second = 360 revolutions / 60 seconds = 6 revolutions/second
- Convert one revolution to radians:
- 1 revolution = 2π radians
- Calculate radians per second:
- Radians per second = 6 revolutions/second * 2π radians/revolution = 12π radians/second
Therefore, the wheel turns through 12π radians in one second.
4. Find the degree measure of the angle subtended at the centre of a circle of radius 100 cm by an arc of length 22 cm (Use π=22/ 7).
Ans :
- Radius of the circle (r) = 100 cm
- Arc length (s) = 22 cm
Formula:
- θ (in radians) = s / r
Calculation:
- θ = 22 cm / 100 cm = 0.22 radians
Converting radians to degrees:
- 1 radian = 180° / π
- θ (in degrees) = 0.22 * (180° / π) ≈ 0.22 * (180° * 7/22) ≈ 12.63°
Therefore, the angle subtended at the center of the circle is approximately 12.63 degrees.
5. In a circle of diameter 40 cm, the length of a chord is 20 cm. Find the length of minor arc of the chord.
Ans :
Given:
- Diameter of the circle = 40 cm
- Length of the chord = 20 cm
Steps:
- Find the radius of the circle:
- Radius = Diameter / 2 = 40 cm / 2 = 20 cm
- Determine the type of triangle formed:
- Since the chord is equal to the radius, the triangle formed by the chord and the radii to its endpoints is an equilateral triangle.
- Find the central angle θ:
- Convert 60 degrees to radians: θ = 60 * (π/180) = π/3 radians
- Use the formula for arc length:
- Arc length (s) = r * θ
- s = 20 cm * (π/3) ≈ 20.94 cm
Therefore, the length of the minor arc of the chord is approximately 20.94 cm.
6. If in two circles, arcs of the same length subtend angles 60° and 75° at the centre, find the ratio of their radii.
Ans :
Given:
- Two circles with arcs of equal length.
- One arc subtends an angle of 60° at the center.
- The other arc subtends an angle of 75° at the center.
Formula:
- Arc length (s) = r * θ
- (where r is the radius and θ is the angle in radians)
Approach:
- Since the arc lengths are equal, we can set up an equation using the formula for arc length.
- Let r1 and r2 be the radii of the two circles, respectively.
- Then, we can write:
- r1 * (60° * π/180) = r2 * (75° * π/180)
Simplifying the equation:
- r1 * (π/3) = r2 * (5π/12)
- r1 / r2 = (5π/12) / (π/3)
- r1 / r2 = 5/4
radii of the two circles is 5:4.
7. Find the angle in radian through which a pendulum swings if its length is 75 cm and th e tip describes an arc of length (i) 10 cm (ii) 15 cm (iii) 21 cm
Ans :
θ = s / r
where:
- θ is the angle in radians
- s is the arc length
- r is the radius (length of the pendulum)
In this case, the radius (r) is 75 cm.
(i) When the arc length (s) is 10 cm: θ = 10 cm / 75 cm = 2/15 radians
(ii) When the arc length (s) is 15 cm: θ = 15 cm / 75 cm = 1/5 radians
(iii) When the arc length (s) is 21 cm: θ = 21 cm / 75 cm = 7/25 radians
Therefore, the angles in radians through which the pendulum swings for the given arc lengths are:
(i) 2/15 radians
(ii) 1/5 radians
(iii) 7/25 radians
Exercise 3.2
Find the values of other five trigonometric functions in Exercises 1 to 5.
1. cos x = – 1/ 2 , x lies in third quadrant.
2. sin x = 3/ 5 , x lies in second quadrant.
3. cot x = 4 /3 , x lies in third quadrant.
4. sec x = 13/ 5 , x lies in fourth quadrant.
5. tan x = – 5 /12 , x lies in second quadrant.
Ans :
1. cos x = -1/2, x lies in third quadrant.
- sin x: Since x is in the third quadrant, sin x is negative. Using the Pythagorean identity:
- sin^2 x + cos^2 x = 1
- sin^2 x + (-1/2)^2 = 1
- sin^2 x = 3/4
- sin x = -√3/2
- tan x: tan x = sin x / cos x = (-√3/2) / (-1/2) = √3
- cot x: cot x = 1 / tan x = 1 / √3 = √3/3
- sec x: sec x = 1 / cos x = 1 / (-1/2) = -2
- csc x: csc x = 1 / sin x = 1 / (-√3/2) = -2√3/3
2.
- cos x: Since x is in the second quadrant, cos x is negative. Using the Pythagorean identity:
- sin^2 x + cos^2 x = 1
- (3/5)^2 + cos^2 x = 1
- cos^2 x = 16/25
- cos x = -4/5
- tan x: tan x = sin x / cos x = (3/5) / (-4/5) = -3/4
- cot x: cot x = 1 / tan x = 1 / (-3/4) = -4/3
- sec x: sec x = 1 / cos x = 1 / (-4/5) = -5/4
- csc x: csc x = 1 / sin x = 1 / (3/5) = 5/3
3.
- tan x: tan x = 1 / cot x = 1 / (4/3) = 3/4
- sin x: Since x is in the third quadrant, sin x is negative. Using the Pythagorean identity:
- 1 + tan^2 x = sec^2 x
- 1 + (3/4)^2 = sec^2 x
- sec^2 x = 25/16
- sec x = -5/4
- cos x = 1 / sec x = -4/5
- sin x = tan x * cos x = (3/4) * (-4/5) = -3/5
- csc x: csc x = 1 / sin x = 1 / (-3/5) = -5/3
4.
- cos x: cos x = 1 / sec x = 1 / (13/5) = 5/13
- sin x: Since x is in the fourth quadrant, sin x is negative. Using the Pythagorean identity:
- sin^2 x + cos^2 x = 1
- sin^2 x + (5/13)^2 = 1
- sin^2 x = 144/169
- sin x = -12/13
- tan x: tan x = sin x / cos x = (-12/13) / (5/13) = -12/5
- cot x: cot x = 1 / tan x = 1 / (-12/5) = -5/12
- csc x: csc x = 1 / sin x = 1 / (-12/13) = -13/12
5. tan x = -5/12, x lies in second quadrant.
- cot x: cot x = 1 / tan x = 1 / (-5/12) = -12/5
- sin x: Since x is in the second quadrant, sin x is positive. Using the Pythagorean identity:
- 1 + tan^2 x = sec^2 x
- 1 + (-5/12)^2 = sec^2 x
- sec^2 x = 169/144
- sec x = -13/12
- cos x = 1 / sec x = -12/13
- sin x = tan x * cos x = (-5/12) * (-12/13) = 5/13
- csc x: csc x = 1 / sin x = 1 / (5/13) = 13/5
Find the values of the trigonometric functions in Exercises 6 to 10.
6. sin 765°
7. cosec (– 1410°)
8. tan 19π /3
9. sin (– 11π /3 )
10. cot (– 15π/ 4 )
Ans :
6. sin 765°
- 765° = 2 * 360° + 45°
- Since sin(360° + θ) = sin(θ), we have:
- sin 765° = sin 45° = √2/2
7. cosec (-1410°)
- csc(-1410°) = -csc(1410°) (since cosecant is odd)
- 1410° = 3 * 360° + 330°
- csc(-1410°) = -csc(330°) = -csc(360° – 30°) = -csc(30°) = -2
8. tan (19π/3)
- 19π/3 = 6π + π/3
- Since tan(2π + θ) = tan(θ), we have:
- tan (19π/3) = tan (π/3) = √3
9. sin (-11π/3)
- sin(-11π/3) = -sin(11π/3) (since sine is odd)
- 11π/3 = 3π + 2π/3
- sin(-11π/3) = -sin(2π/3) = -√3/2
10. cot (-15π/4)
- cot(-15π/4) = -cot(15π/4) (since cotangent is odd)
- 15π/4 = 4π + 3π/4
- cot(-15π/4) = -cot(3π/4) = 1
Exercise 3.3
Prove that:
1. sin2 π/6 + cos2 π/3 – tan2 π/4 = – ½
Ans :
Given: sin²(π/6) + cos²(π/3) – tan²(π/4) = -1/2
Solution:
Let’s calculate each term separately:
- sin²(π/6): The sine of π/6 (30 degrees) is 1/2. So, sin²(π/6) = (1/2)² = 1/4.
- cos²(π/3): The cosine of π/3 (60 degrees) is also 1/2. So, cos²(π/3) = (1/2)² = 1/4.
- tan²(π/4): The tangent of π/4 (45 degrees) is 1. So, tan²(π/4) = 1².
Substituting these values into the original equation:
(1/4) + (1/4) – 1 = -1/2 1/2 – 1
= -1/2 -1/2 = -1/2
Conclusion: The left side of the equation equals the right side. Therefore, the identity is proven:
sin²(π/6) + cos²(π/3) – tan²(π/4)
= -1/2
2. sin2 π/6 + cosec2 7π/6 cos2 π/3 = 3/2
Ans :
3. cot2 π/6 + cosec 5π/6 + 3 tan2 π/6 = 6
Ans :
1. cot²(π/6):
- cot(π/6) = 1 / tan(π/6)
- = 1 / (1/√3)
- = √3
- cot²(π/6) = (√3)² = 3
2. cosec(5π/6):
- 5π/6 is in the second quadrant, where cosecant is positive.
- cosec(5π/6) = 1 / sin(5π/6) = 1 / (1/2) = 2
3. tan²(π/6):
- tan(π/6) = 1/√3
- tan²(π/6) = (1/√3)² = 1/3
3 + 2 + 3 * (1/3) = 6
Simplifying:
3 + 2 + 1 = 6 6 = 6
Therefore, the given identity is true.
4. 2 sin2 3π/4 + 2 cos2 π/4 + 2 sec2 π/3 = 10
Ans :
1. sin²(3π/4):
- sin(3π/4) = 1/√2
- sin²(3π/4) = (1/√2)² = 1/2
2. cos²(π/4):
- cos(π/4) = 1/√2
- cos²(π/4) = (1/√2)² = 1/2
3. sec²(π/3):
- sec(π/3) = 1 / cos(π/3) = 1 / (1/2) = 2
- sec²(π/3) = 2² = 4
2 * (1/2) + 2 * (1/2) + 2 * 4 = 10
Simplifying:
1 + 1 + 8 = 10 10 = 10
Therefore, the given identity is true.
5. Find the value of:
(i) sin 75° (ii) tan 15°
Ans :
1. sin 75°
- 75° = 150°/2
- cos 150° = -√3/2 (since 150° is in the second quadrant)
Therefore,
sin 75° = √[(1 – (-√3/2))/2] = √[(2 + √3)/4] = (√6 + √2)/4
2. tan 15°
- 15° = 30°/2
- cos 30° = √3/2
Therefore,
tan 15° = √[(1 – √3/2)/(1 + √3/2)] = √[(2 – √3)/(2 + √3)] = 2 – √3
So, the values are:
(i) sin 75° = (√6 + √2)/4 (ii) tan 15° = 2 – √3
Prove the following:
6. cos(π/4−x)cos(π/4−y)−sin(π/4−x)sin(π/4−y) = sin (x + y)
Ans :
Given: cos(π/4−x)cos(π/4−y)−sin(π/4−x)sin(π/4−y) = sin (x + y)
Proof:
We will use the following trigonometric identity:cos(A + B) = cosAcosB – sinAsinB
In our case, we have:
- A = π/4 – x
- B = π/4 – y
- cos((π/4 – x) + (π/4 – y))
Simplifying:
- cos(π/2 – (x + y))
Using the identity cos(π/2 – θ) = sin θ, we get:
- sin(x + y)
7. tan(π/4+x)/tan(π/4−x)=(1+tanx1−tanx)2
Ans :
tan(A + B) = (tan A + tan B) / (1 – tan A * tan B)
In this case, A = π/4 and B = x.
Substituting into the formula:
tan(π/4 + x) = (tan(π/4) + tan x) / (1 – tan(π/4) * tan x)
Since tan(π/4) = 1, we get:
tan(π/4 + x) = (1 + tan x) / (1 – tan x)
Now, we can rewrite the original identity as:
(tan(π/4 + x) / tan(π/4 – x)) = (1 + tan x / 1 – tan x)²
Substituting the expression for tan(π/4 + x):
((1 + tan x) / (1 – tan x)) / (tan(π/4 – x)) = (1 + tan x / 1 – tan x)²
Since tan(π/4 – x) = (1 – tan x) / (1 + tan x) (using the tangent subtraction formula), we can substitute this into the equation:
((1 + tan x) / (1 – tan x)) / ((1 – tan x) / (1 + tan x)) = (1 + tan x / 1 – tan x)²
Simplifying the left side:
(1 + tan x)² / (1 – tan x)² = (1 + tan x / 1 – tan x)²
Therefore, the identity is proven.
8. cos(π+x)cos(−x)/sin(π−x)cos(π/2+x) = cot2 x
Ans :
To prove the given identity, we will use the following trigonometric identities:
- cos(π + x) = -cos(x)
- cos(-x) = cos(x)
- sin(π – x) = sin(x)
- cos(π/2 + x) = -sin(x)
Substituting these identities into the given equation, we get:
(-cos(x)) * cos(x) / (sin(x) * (-sin(x))) = cot² x
Simplifying the expression:
cos²(x) / sin²(x) = cot² x
Since cot x = cos x / sin x, we can rewrite the left side as:
(cos x / sin x)² = cot² x
Therefore, the given identity is proven.
9.
Ans :
=1= R.H.S
10. sin (n + 1)x sin (n + 2)x + cos (n + 1)x cos (n + 2)x = cos x
Ans :
cos(A + B) = cos A cos B – sin A sin B
In this case, let A = (n + 1)x and B = (n + 2)x.
Substituting into the identity:
cos((n + 1)x + (n + 2)x) = cos(n + 1)x cos(n + 2)x – sin(n + 1)x sin(n + 2)x
Simplifying the left side:
cos(2n + 3)x = cos(n + 1)x cos(n + 2)x – sin(n + 1)x sin(n + 2)x
Now, we can rearrange the terms to match the given identity:
sin(n + 1)x sin(n + 2)x + cos(n + 1)x cos(n + 2)x = cos(2n + 3)x
Since cos(2n + 3)x is equal to cos(x) for all integer values of n (due to the periodicity of the cosine function), we have:
sin(n + 1)x sin(n + 2)x + cos(n + 1)x cos(n + 2)x = cos x
Therefore, the identity is proven.
11. cos(3π/4+x)−cos(3π/4−x)=−2–√sinx
Ans :
cos(A – B) – cos(A + B) = 2sin A sin B
let A = 3π/4 and B = x.
Substituting into the identity:
cos(3π/4 – x) – cos(3π/4 + x) = 2sin(3π/4)sin(x)
Since sin(3π/4) = √2/2, we can substitute this value into the equation:
cos(3π/4 – x) – cos(3π/4 + x) = 2(√2/2)sin(x)
Simplifying:
cos(3π/4 – x) – cos(3π/4 + x) = √2sin(x)
Therefore, the given identity is proven.
12. sin2 6x – sin2 4x = sin 2x sin 10 x
Ans :
To prove this identity, we will use the following trigonometric identity:
sin² A – sin² B = sin(A + B)sin(A – B)
Substituting into the identity:
sin²(6x) – sin²(4x) = sin(6x + 4x)sin(6x – 4x)
Simplifying:
sin²(6x) – sin²(4x) = sin(10x)sin(2x)
Therefore, the given identity is proven.
13. cos2 2x cos2 6x = sin 4x sin 8x
Ans :
sin(2A) = 2sin(A)cos(A)
We can rewrite the given identity as:
2sin(4x)cos(4x) = sin(8x)sin(2x)
Now, using the identity sin(2A) = 2sin(A)cos(A) for both terms:
2(2sin(2x)cos(2x)) = 2sin(4x)sin(2x)
Simplifying:
4sin(2x)cos(2x) = 2sin(4x)sin(2x)
Dividing both sides by 2sin(2x):
2cos(2x) = sin(4x)
Since sin(4x) = 2sin(2x)cos(2x),
the given identity is proven.
14. sin 2x + 2 sin 4x + sin 6x = 4 cos2 x sin 4x
Ans :
L.H.S.= sin 2x + 2 sin 4x + sin 6x
= [sin 2x + sin 6x] + 2 sin 4x
= [2sin(2x+6×2)cos(2x−6×2)] + 2 sin 4x
[∵ sin A + sin B = 2 sin(A+B2)cos(A−B2)
= 2 sin 4x cos (- 2x) + 2 sin 4x
= 2 sin 4x cos 2x + 2 sin 4x
= 2 sin 4x (cos 2x + 1)
= 2 sin 4x (2 cos2 x – 1 + 1)
= 2 sin 4x (2 cos2 x)
= 4 cos2 x sin 4x
= R.H.S.
Hence proved.
15. cot 4x (sin 5x + sin 3x) = cot x (sin 5x – sin 3x)
Ans :
16. Cos9x−cos5x / sin17x−sin3x =−sin2x / cos10x
Ans :
17. Sin5x+sin3x / cos5x+cos3x = tan 4x
Ans :
sin(A) + sin(B) = 2sin((A + B)/2)cos((A – B)/2)
cos(A) + cos(B) = 2cos((A + B)/2)cos((A – B)/2)
Substituting A = 5x and B = 3x into these formulas:
sin(5x) + sin(3x) = 2sin((5x + 3x)/2)cos((5x – 3x)/2) = 2sin(4x)cos(x)
cos(5x) + cos(3x) = 2cos((5x + 3x)/2)cos((5x – 3x)/2) = 2cos(4x)cos(x)
Now, we can substitute these expressions into the given identity:
(sin(5x) + sin(3x)) / (cos(5x) + cos(3x)) = (2sin(4x)cos(x)) / (2cos(4x)cos(x))
Simplifying:
(sin(4x)cos(x)) / (cos(4x)cos(x)) = sin(4x) / cos(4x)
Since tan(x) = sin(x) / cos(x), we have:
sin(4x) / cos(4x) = tan(4x)
Therefore, the given identity is proven.
18. sinx−siny/cosx+cosy =tanx−y/2
Ans :
.
19. Sinx+sin3x / cosx+cos3x = tan 2x
Ans :
20. Sinx−sin3x / sin2x−cos2x = 2 sin x
Ans :
It is known that
sin A – sin B = 2 cos(A+B /2)
sin(A−B/ 2)cos2 A – sin2 A = cos 2A
∴ L.H.S. = sinx−sin3x / sin2x−cos2x
= 2cos(x+3x/2)sin(x−3x/2)/ 2cos2x
= – 2 × (- sin x) = 2 sin x
= R.H.S
Hence proved.
21. Cos4x+cos3x+cos2x / sin4x+sin3x+sin2x= cot 3x
Ans :
22. cot x cot 2x – cot 2x cot 3x – cot 3x cot x = 1
Ans :
L.H.S.= cot x cot 2x – cot 2x cot 3x – cot 3x cot x
= cot x cot 2x – cot 3x (cot 2x + cot x)
= cot x cot 2x – cot (2x + x) (cot 2x + cot x)
= cot x cot 2x – [cot2xcotx−1 / cotx+cot2x] (cot 2x + cot x)
[∵ cot (A + B) = cotAcotB−1 / cotA+cotB]
= cot x cot 2x – (cot 2x cot x – 1)
= 1 = R.H.S.
Hence proved.
23. tan 4x = 4tanx(1−tan2x) / 1−6tan2x+tan4x
Ans :
.
24. cos 4x = 1 – 8sin2 x cos2 x
Ans :
To prove the given identity, we will use the following trigonometric identities:
cos(2A) = 1 – 2sin²(A) sin(2A) = 2sin(A)cos(A)
Substituting A = 2x into the first identity:
cos(4x) = 1 – 2sin²(2x)
Now, using the identity sin(2A) = 2sin(A)cos(A) with A = x:
sin²(2x) = (2sin(x)cos(x))²
Substituting this into the previous equation:
cos(4x) = 1 – 2(2sin(x)cos(x))²
Simplifying:
cos(4x) = 1 – 8sin²(x)cos²(x)
Therefore, the given identity is proven.
25. cos 6x = 32 cos6 x – 48cos4 x + 18 cos2 x – 1
Ans :
To prove this identity, we will use the double angle formula for cosine:
cos(2A) = 2cos²(A) – 1
We can start by expressing cos(6x) in terms of cos(2x) using this formula:
cos(6x) = cos(2(3x)) = 2cos²(3x) – 1
Now, we can use the double angle formula again to express cos²(3x) in terms of cos(6x):
cos²(3x) = (1 + cos(6x))/2
Substituting this into the previous equation:
cos(6x) = 2((1 + cos(6x))/2) – 1
Simplifying:
cos(6x) = 1 + cos(6x) – 1
cos(6x) = cos(6x)
This is a tautology, meaning it is always true. Therefore, the given identity is proven.